How To Get A Common Denominator
Least Common Denominator Calculator
Find the least common denominator for a set of fractions by providing a list of denominators below.
Solution:
The least common denominator of 22, 88, & 132 is 264
Steps to Solve
264 is the smallest number that is evenly divisible by 22, 88, & 132 without a remainder
264 ÷ 22 = 12
264 ÷ 88 = 3
264 ÷ 132 = 2
How to Find the Least Common Denominator
A denominator is the bottom number of a fraction, or the number below the fraction line. For a fraction 1/3, the denominator is 3.
A common denominator is a denominator that is common to the fractions being operated on. For the denominator to be common it must be the same in all fractions.
For instance, 1 3 and 2 3 have common denominators – the denominators are the same.
1 3 and 2 5 do not have common denominators – the denominators are different.
The least common denominator is the smallest common denominator. It is the smallest whole number that is evenly divisible by all uncommon denominators. The least common denominator is also referred to as the lowest common denominator or the least common multiple.
The least common denominator for the fractions 1 3 and 2 5 is 15.
15 ÷ 3 = 5
15 ÷ 5 = 3
Note that there can be no remainder when dividing by one of the denominators by the least common denominator.
There are a few methods that can be used to find the least common denominator. The easiest is to use the calculator above. If you want to do the work yourself, follow along to learn two methods to solve it.
Method One: Find the Least Common Denominator Using Factorization
One way to find the least common denominator is to use prime factorization. Find the prime factors of each denominator. Then multiply all of the prime factors, multiplying the factors that are common to both only once, to find the least common denominator.
You'll probably find our factors calculator helpful to find the factors of your denominators, including the greatest common factor.
Example 1: Find the Least Common Denominator of 90 and 36
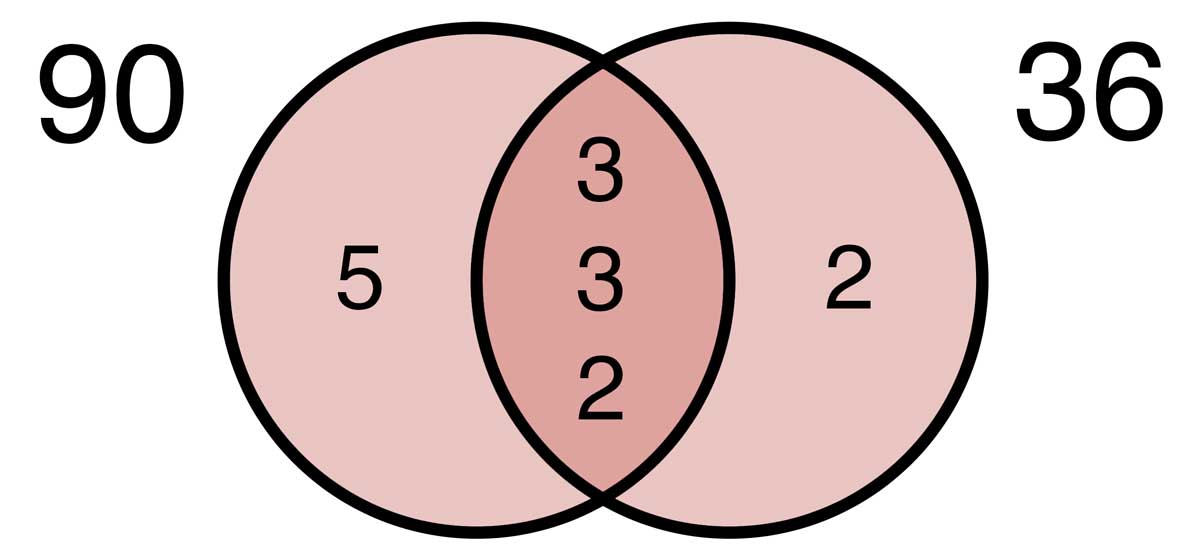
Step one: find the prime factors of 36.
Divide 36 by 2, which equals 18. 18 and 2 are factors. 18 can be factored again into 6 and 3. 6 can be factored into 2 and 3. The prime factors are thus [3, 3, 2, 2].
Step two: find the prime factors of 90.
Divide 90 by 10, which equals 9. 9 and 10 are factors. 9 can be factored again into 3 and 3. 10 can be factored into 5 and 2. The prime factors are thus [5, 3, 3, 2].
Step three: find the prime factors that are common to both 36 and 90.
The prime factors of 36 and 90 are [5, 3, 3, 2, 2].
Step four: multiply the factors together to find the least common denominator. Note that since 3, 3, and 2 are common between 36 and 90 they are only used once.
LCD = 5 × 3 × 3 × 3 × 2 × 2
LCD = 180
Example 2: Find the Least Common Denominator of 105 and 165
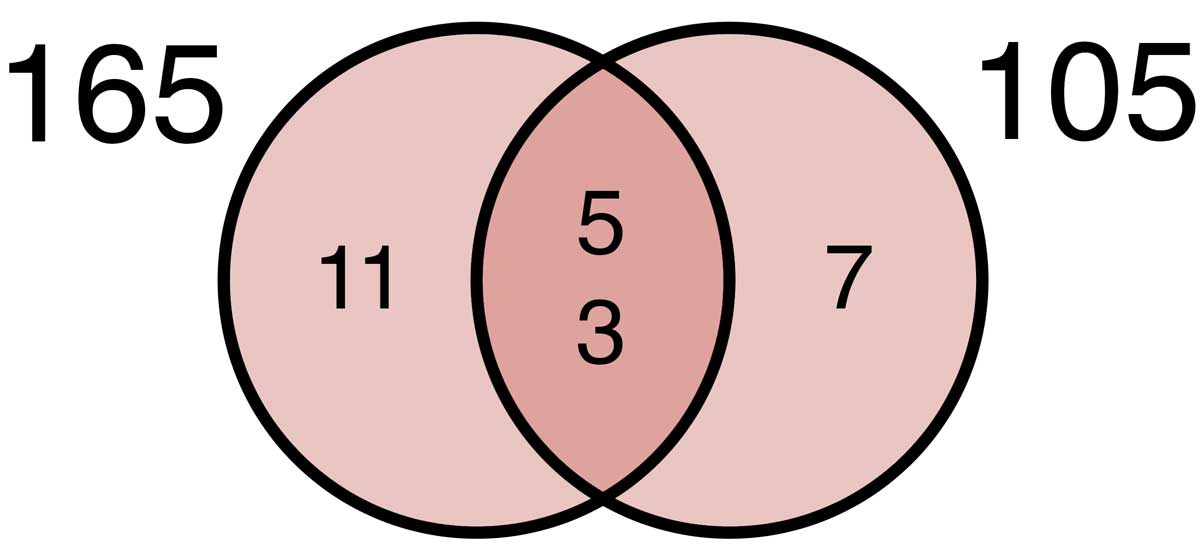
Step one: find the prime factors of 105.
Divide 105 by 7, which equals 15. 15 and 7 are factors. 15 can be factored again into 5 and 3. The prime factors are thus [7, 5, 3].
Step two: find the prime factors of 165.
Divide 165 by 11 which equals 15. 15 and 11 are factors. 15 can be factored again into 5 and 3. The prime factors are thus [11, 5, 3].
Step three: find the prime factors that are common to both 105 and 165.
The prime factors of 105 and 165 are [11, 7, 5, 3].
Step four: multiply the factors together to find the least common denominator. Note that 5 and 3 are common between 105 and 165 so they are only used once.
LCD = 11 × 7 × 5 × 3
LCD = 1,155
Example 3: Find the Least Common Denominator of 24 and 42
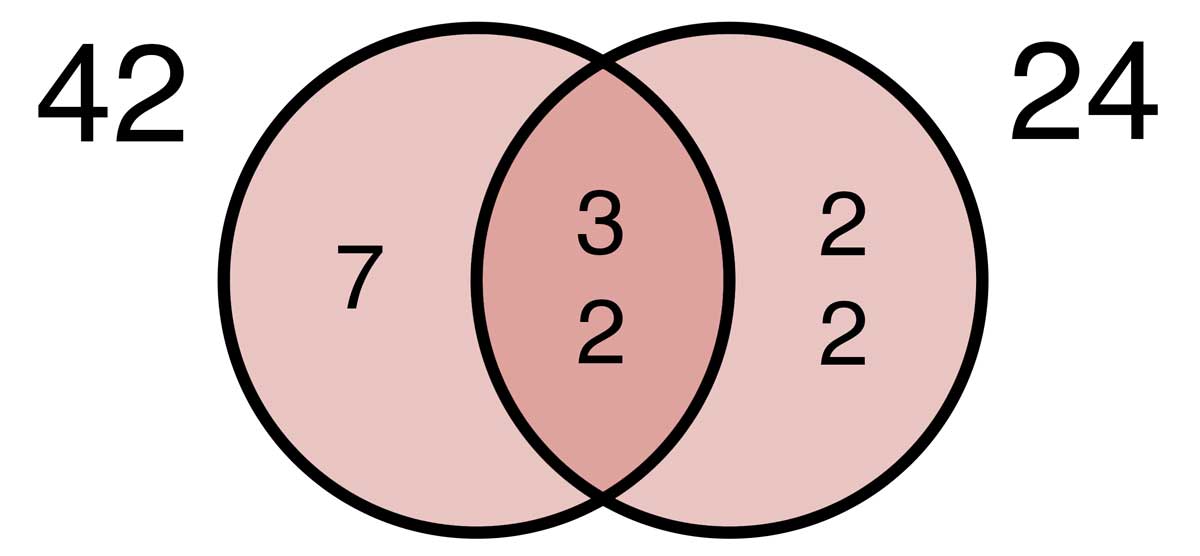
Step one: find the prime factors of 24.
Divide 24 by 2, which equals 12. 12 and 2 are factors. 12 can be factored again into 6 and 2. 6 can be factored again into 2 and 3. The prime factors are thus [3, 2, 2, 2].
Step two: find the prime factors of 42.
Divide 42 by 7 which equals 6. 7 and 6 are factors. 6 can be factored again into 2 and 3. The prime factors are thus [7, 3, 2].
Step three: find the prime factors that are common to both 24 and 42.
The prime factors for 24 and 42 are [7, 3, 2, 2, 2].
Step four: multiply the factors together to find the least common denominator. Note that 3 and 2 are common between 24 and 42 so they are only used once.
LCD = 7 × 3 × 2 × 2 × 2
LCD = 168
Method Two: Find the Least Common Denominator by Finding all Multiples
You can also find the least common denominator by finding all of the multiples of each denominator and finding the smallest multiple that is common to both.
To find multiples of a number, multiply it by 2 to find the first multiple. Then multiply it by 3 to find the second. Continue by multiplying it by 4, and so on to find all of the multiples.
For example, let's find the least common denominator of 3 and 5 by finding all multiples.
The multiples of 3 are [3,6,9,12,15,18,21,24,27,30,…]
The multiples of 5 are [5,10,15,20,25,30,…]
Observe that the multiples that are common to both 3 and 5 are 15 and 30. The smallest of these is 15, which makes it the least common denominator.
Thus, the least common denominator of 3 and 5 is 15.
How To Get A Common Denominator
Source: https://www.inchcalculator.com/least-common-denominator/
Posted by: smithvitioneste.blogspot.com

0 Response to "How To Get A Common Denominator"
Post a Comment